Lentil convexity

|
Author’s note. I mean no slight on people who buy tinned lentils. I, personally, quite like them.
So it turns out we haven’t been panic hoarding lentils after all. There is a benign explanation for the sudden disappearance of split peas from the nation’s grocery shelves.
And it is all to do with when seemingly normal distributions reveal themselves to be leptokurtic. Yes, you read that right. This is all about kurtosis: the measure of distribution of improbable events. Fat tails.
In ordinary times, our lentil-buying habits are regular: hippies and vegans (a small (but growing!) portion of the population) buy a lot of lentils, and everyone else buys none. Okay, almost none. The person on the Clapham Omnibus might have one tin, at the back of the cupboard, that someone got in a weak moment years ago, just in case of unexpected apocalypse, or an unexpected visit from your long-lost, vegan, cousin from Australia.
But the peacetime lentil-buying motivations of a hippy, a vegetarian and the proverbial meat-and-potatoes munching, Clapham omnibus-riding ordinary fellow are quite distinct, and unrelated. Each person’s decisions are, within a fairly tight range, predictable and and independent of each other: my lentil acquisition does not greatly influence, and is not particularly correlated with, yours.
National weekly lentil purchases therefore usually cleave to a normal distribution. A small part of the population (say 0.5% - the hippies) may buy 8, 9 or 10 tins. A larger part (say 4.5% - vegans, health-food fanatics etc.) may buy one or two, and the remaining 95% will buy very few (lets’ say on average 0.1 tin each: one tin between ten, which is probably generous). Since lentil buyers’ decisions are unconnected — that is the acquisition of a tin of lentils is an independent event[1] — it all tends to even out, within a range. Each of these numbers will fluctuate, week to week, but in the same week that your hippie stocks up extra for his bom shankar summer solstice druid’s convention cauldron partay, your vegan might skip a tin, and your 90% who hardly ever buy lentils make little difference to the acquisition rate in any weather.
The odds of everyone, including the normals, all going large on lentils in the same week is extremely low.[2] The consequence, across the community, is a normal distribution of weekly lentil acquisition. The random variation in purchases by people in the different demographic groups will cause a small fluctuation in in demand for lentils from week to week, but from a grocer’ perspective, the demand curve is predictable and manageable.
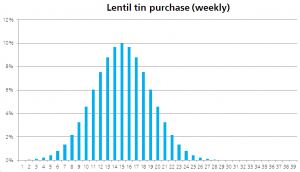
But in actual fact lentil purchasing decisions are not independent, as our new friend the coronavirus pandemic illustrates. They just seem like it, in most markets. 95 percent of the sample are connected by a general disposition not to buy lentils. But this disposition is a function of one’s apprehension of the proximity of apocalypse. Apocalypses are, in the main, rare, and one does not tend to form an opinion that one is nigh purely through ones’ own deductions from meteorological and astronomical data. Rather, we apprehend oblivion because someone said it on Twitter. Because Owen Jones wrote a thought-piece on it, or because — by Jupiter, everyone is suddenly buying lentils and there are hardly any left in Sainsbury’s. We get infected wioth the idea of apocaluyopse from our fellow citizens.
In other words, our legume-buying habits are not independent after all. Lentil purchases are not independent. They are all formed on a consensus about the non-imminence of the second coming. While each person’s threshold for precautionary lentil purchase in the event of imminent apocalypse will differ, across the group, news of the unchecked spread of coronavirus will bring each person closer to that threshold, and some of them over it.
As they walk past the tinned goods shelf, it only takes a small proportion of that 95% to pick up a tin to blow the grocer's expectations out the window. Let’s say 5 of the 95% decide to buy a in each.assume also that the hippies vegans and health food fanatics in the sample are also buying their regular quota, we can see that the grocers supply of lentils will quickly be depleted.
And now a second order of dependence emerges. For imagine some of the 95% who have not crossed the the threshold for precautionary lentil purchase, but notice that the lentil shelf in the supermarket is nearly empty. This might prompt them to reconsider there apprehension of apocalypse. They will collect the remaining lentils.
The supermarket management will now intervene, alarmed at this sudden run on lentils. There immediate reaction is to impose an item limit. a sign is posted on the shelf prominently restricting customers to 3 tins each.
Now remaining members of the 95% could hardly have asked for a clearer sign of of armageddon than LENTIL RATIONING — I mean, panic buying hippy food: could it get any worse than that? Seeing as it is too late to stock up on lentils, they head to the tinned borlotti beans.
But to their horror they discover the borlotti beans have been cleaned out as well. Their suspicions about forthcoming apocalypse now confirmed, they stampede towards the couscous and quinoa.
By day 3 there is a nervous scrum of people standing at the the empty shelves waiting for replenishment of tinned lentils.
See also
References
- ↑ BUT IS IT. I don’t want to spoil the punchline but HOLD THAT THOUGHT.
- ↑ With genuinely unconnected events the probabilities fade into cosmic radiation fast: the odds of tossing heads just 100 times in a row with a fair coin is one in half a googol. If it takes ten minutes to toss 100 times, you wouldn’t expect it to happen in several lives of the universe.